Derivative of Volume of a Cylinder With Respect to Radius

Height of a Triangle. Height of a Cylinder.

Volume Of Cylinder In Terms Of Pi Formula Examples Definition

As much as you want this to be a trick question you will not get the solution unless you write THE FORMULA for the volume of a cylinder.

. Homogeneous System of Equations. Height of a Pyramid. Dr dt 1 dh dt 4 Goal.

Height of a Prism. A cylinder is leaking water but you are unable to determine at what rate. Find the rate at which the water is leaking out of the cylinder if the rate at which the height is decreasing is 10 cmmin when the height is 1 m.

What is the rate of change of the volume of the cylinder at the instant. When we find the partial derivative π r 2 h r fracpartialpi r2hpartialr r π r 2 h we find the rate of change of the cylinders volume as only the radius changes. The cylinder has a height of 2 m and a radius of 2 m.

Find dV dt when r 5 h 8. This function represents the volume of a cylinder. You have a product rule on.

Height of a Parallelogram. We use the volume formula for a cylinder V ˇr2h Di erentiate both sides with respect to t. Height of a Trapezoid.

Certain instant the base radius is 5 meters and the height is 8 meters.

101 Maximum Volume Of Cylinder With Surface Area 384 Pi Derivatives Calculus Youtube

Relationship Between Volume Of A Cylinder And A Sphere Youtube

This Page Examines The Properties Of A Right Circular Cylinder A Cylinder Has A Radius R And A Height H See Picture Below Solid Geometry Cylinder Volume

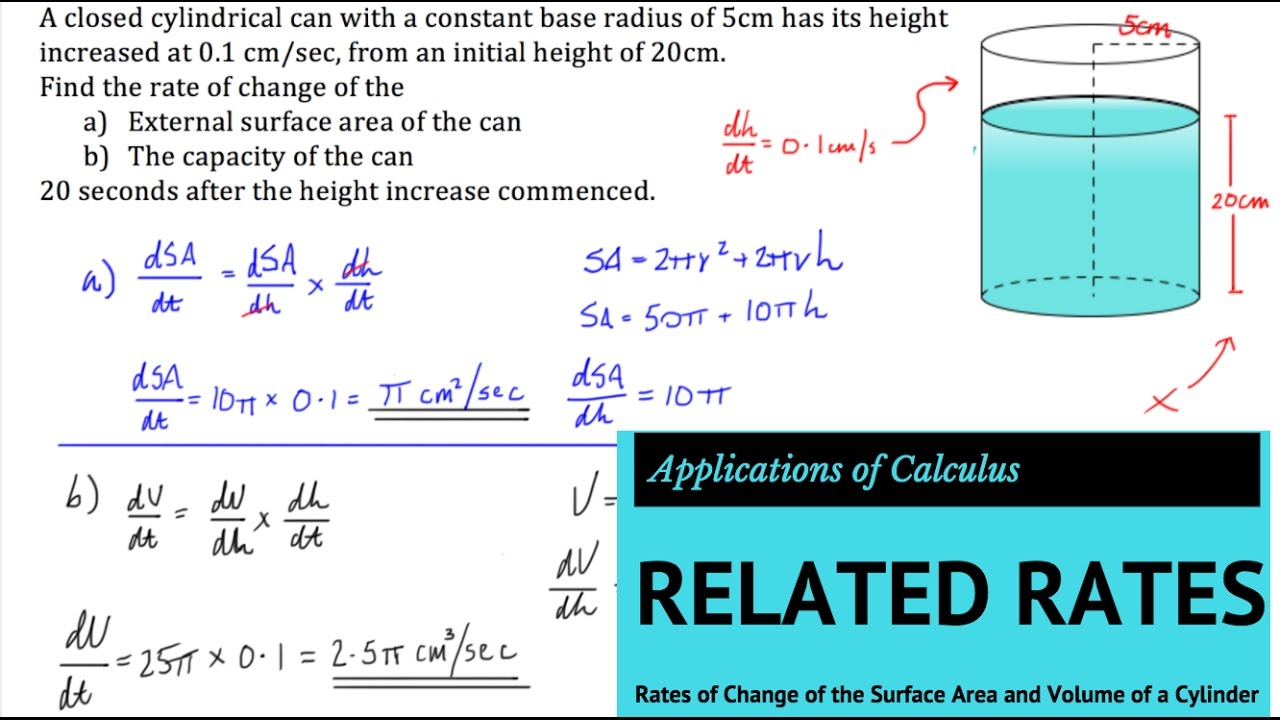
Related Rates Finding The Rates Of Change Of Surface Area And Volume Of A Cylinder Youtube